COMPARATIVE ANALYSIS OF PLASMA-CHEMICAL MODELS FOR COMPUTER SIMULATION OF GLOW DISCHARGES IN AIR MIXTURES

Subject of Research.  We present research results for numerical modeling possibility of discharges in gas mixtures within the modern model of nonlocal plasma by creation a sequence of plasma-chemical and numerical models and comparing the results with experimental data. Method. Creation method for series of models with gradually increasing complexity has been used. It is based on a step by step expansion of the range accounted for elementary processes in nonlocal glow discharge plasma in the air. The air is approximated by the mixture of nitrogen and oxygen at low pressures under conditions suitable for experimental verification. For each iteration of plasma chemical scheme, corresponding numerical models of gas-discharge were created. The graphs of the discharge gap electrical parameters on the pressure were obtained by this method. Theoretical data obtained at each step have been compared to the experimental data and the results of previous computer models. Main Results. The model has been created  that provides a good agreement with the experimentally obtained dependencies of the voltage drop across the discharge gap on the gas pressure in the areas of normal and abnormal glow discharge. By the updated model the optimum value for the coefficient of secondary electron emission from the cathode was chosen. Additionally, we have obtained the spatial distribution of the internal parameters of nonlocal plasma (longitudinal and transverse profiles of the electric potential, electron and ion densities, the electron temperature) as a subject to further experimental verification. Practical Relevance. The created models are perspective to be used for diagnosis and the setting of parameters of micro-discharges in the air. They have different applications, including developing method of electronic collision spectroscopy (CES) gas mixtures.

1. Kudryavtsev A.A., Smirnov A.S., Tsendin L.D. Fizika Tleyushchego Razryada [Physics of Glow Discharge]. St. Petersburg, Lan' Publ., 2010, 512 p.
2. Granovskii V.L. Elektricheskii Tok v Gaze. Ustanovivshiisya Tok [Electric Current in the Gas. Sustained Current]. Moscow, Nauka Publ., 1971, 490 p.
3. Raizer Yu.P. Fizika Gazovogo Razryada [Gas Discharge Physics]. Moscow, Nauka Publ., Fizmatlit Publ., 1987, 592 p.
4. Bogdanov E.A., Kapustin K.D., Kudryavtsev A.A., Chirtsov A.S. Different approaches to fluid simulation of the longitudinal structure of the atmospheric-pressure microdischarge in helium. Technical Physics. The Russian Journal of Applied Physics, 2010, vol. 55, no. 10, pp. 1430–1442. doi: 10.1134/S1063784210100063
5. Kapustin K., Kudryavtsev A., Bogdanov E., Chirtsov A. Nonlocal behavior of electron fluxes and excitation rates for “local” EEDF in moderate and high pressures DC positive column plasmas. IEEE Transactions on Plasma Science, 2011, vol. 39, no. 11, pp. 2580–2581. doi: 10.1109/TPS.2011.2140385
6. Eliseev S.I., Demidov V.I., Chirtsov A.S., Kudryavtsev A.A., Kolobov V.I., Bogdanov E.A. Simulation of pulsed breakdown in helium by adaptive methods. Scientific and Technical Journal of Information Technologies, Mechanics and Optics, 2014, no. 5, pp. 139–146.
7. Bogdanov E.A., Chirtsov A.S., Kudryavtsev A.A. Fundamental nonambipolarity of electron fluxes in 2D plasmas. Physical Review Letters, 2011, vol. 106, no. 19, art. 195001. doi: 10.1103/PhysRevLett.106.195001
8. Kaganovich I.D., Demidov V.I., Adams S.F. Raitses Y. Non-local collisionless and collisional electron transport in low-temperature plasma. Plasma Physics and Controlled Fusion, 2009, vol. 51, no. 12, art. 124003. doi: 10.1088/0741-3335/51/12/124003
9. Comsol introduction. Humusoft. Available at: www.humusoft.com/comsol (accessed 30.08.2016).
10. Ashihara O. The electron energy loss rates by polar molecules. Tokyo, Institute of Space and Aeronautical Science, 1975, vol. 40, no. 530, pp. 257.
11. Dalidchik F.I., Sayasov Yu.S. Recombination of electrons in molecular gases. Journal of Experimental and Theoretic Physics, 1965, vol. 49, pp. 302–305.
12. Rudge M.R.H. Theory of ionization of atoms by electron impact. Reviews of Modern Physics, 1968, vol. 40, pp. 564. doi: 10.1103/RevModPhys.40.564
13. Biagi Database. Plasma Data Exchange Project. Available at: www.lxcat.net/Biagi (accessed 22.04.2016).
14. Morgan (Kinema Research & Software) Database. Available at: www.lxcat.net/Morgan (accessed 22.04.2016).
15. Phelps Database IST-Lisbon Database. Available at: www.lxcat.net/Phelps (accessed 22.04.2016).
16. Vainshtein L.A., Sobel'man I.I., Yukov E.A. Secheniya Vozbuzhdeniya Atomov i Ionov Elektronami [Excitation Cross Sections of Atoms and Ions by Electrons]. Moscow, Nauka Publ., 1973, 142 p.
17. Fok V.A. Nachala Kvantovoi Mekhaniki [Principles of Quantum Mechanics]. Moscow, Nauka Publ., 1976, 376 p.
18. Sobel'man I.I. Vvedenie v Teoriyu Atomnykh Spektrov [Introduction to the Theory of Atomic Spectra]. Moscow, Fizmatgiz Publ., 1963, 640 p.
19. Sychov S., Chirtsov A. Genetic algorithm as a means for solving a radial Schrödinger equations system. Proc. XIX Int. Conf. on Soft Calculations and Measurements.
St.-Petersburg, 2003, pp. 489–492.
20. Tuhvatulin A.I., Sysolyatina E.V., Scheblyakov D.V. et. al. Non-thermal plasma causes P53-depended apoptosis in human colon carcinoma cells. Acta Naturae, 2012, vol. 4, no. 3, pp. 82–87.
21. Stroikova I.K., Maksimov A.I. Disinfecting of solutions by glow and diaphragm discharges at atmospheric pressure. Elektronnaya Obrabotka Materialov, 2002, vol. 38, no. 6, pp. 43–49. (In Russian)
22. Kalinin L.G., Panchenko G.I., Boshkova I.L., Kolomiichuk S.G. Influence of low-frequency and microwave electromagnetic fields on seeds. Biophysics, 2005, vol. 50, no. 2, pp. 334–337.
23. Kudryavtsev A.A., Chirtsov A.S., Yakovleva V.I., Mustafaev A.S., Tsyganov A.B. Electron energy spectra in helium observed in a microplasma collisional electron spectroscopy detector. Technical Physics. The Russian Journal of Applied Physics, 2012, vol. 57, no. 10, pp. 1325–1330. doi: 10.1134/S1063784212100106
24. DeJoseph Jr., C.A., Demidov V.I., Kudryavtsev A.A. Nonlocal effects in a bounded low-temperature plasmas with fast electrons. Physics of Plasmas, 2007, vol. 14, no. 5, art. 057101. doi: 10.1063/1.2436470
25. Bogdanov E.A., Kudryavtsev A.A., Ochikova Z.S. Main scenarios of spatial distribution of charged and neutral components in SF6 plasma. IEEE Transactions on Plasma Science, 2013, vol. 41, no. 12, pp. 3254–3267. doi: 10.1109/TPS.2013.2278839
26. Walker J.J., Koepke M.E., Zimmerman M.I., Farrell W.M., Demidov V.I. Analytical model for gyro-phase drift arising from abrupt inhomogeneity. Journal of Plasma Physics, 2014, vol. 80, no. 3, pp. 395–404. doi: 10.1017/S0022377813001359
27. Bogdanov E.A., Demidov V.I., Kaganovich I.D., Koepke M.E., Kudryavtsev A.A. Modeling a short DC discharge with thermionic cathode and auxiliary anode. Physics of Plasmas, 2013, vol. 20, no. 10, art. 101605. doi: 10.1063/1.4823464
28. Koepke M.E., Walker J.J., Zimmerman M.I., Farrell W.M., Demidov V.I. Signature of gyro-phase drift. Journal of Plasma Physics, 2013, vol. 79, no. 6, pp. 1099–1105. doi: 10.1017/S0022377813001128
29. Astafiev A.M., Gutsev S.A., Kudryavtsev A.A. Study of the discharge with an electrolytic electrode (Gatchina’s discharge). Vestnik St. Petersburg State University. Ser. 4. Phys. Chem, 2013, no. 4, pp. 139–142.
30. Barzilovich K.A., Bogdanov E.A., Kudryavtsev A.A.
A criterion of field reversal in a short glow discharge and its dependence on the parameters of the fluid model used. Technical Physics Letters, 2014, vol. 40, no. 7, pp. 581–583. doi: 10.1134/S1063785014070025
31. Bogdanov E.A., Demidov V.I., Kudryavtsev A.A., Saifutdinov A.I. Is the negative glow plasma of a direct current glow discharge negatively charged? Physics of Plasmas, 2015, vol. 22, no. 2, art. 024501. doi: 10.1063/1.4907192
32. Bogdanov E.A., Kudryavtsev A.A., Ochikova Z.S., Chirtsov A.S. Violation of the boltzmann distribution for plasma electron number density in two-chamber inductively coupled plasma discharges. Technical Physics, 2015, vol. 60, no. 10, pp. 1570–1573. doi: 10.1134/S1063784215100084
33. Chen Z., Yin Z., Huang Y., Stepanova O.M., Gutsev S.A., Kudryavtsev A.A. More efficient microwave argon plasma jet with a symmetric hairpin copper wire at atmospheric pressure. IEEE Transactions on Plasma Science, 2015, vol. 43, no. 3, pp. 906–907. doi: 10.1109/TPS.2015.2393552
34. Tchernycheva M.V., Marek V.P., Chirtsov A.S., Shvager D.A. Computational modeling in the study of glow discharge physical processes in the air at low pressures. Scientific and Technical Journal of Information Technologies, Mechanics and Optics, 2014, no. 3, pp. 140–148. (In Russian)
35. Raizer Yu.P. High-frequency high-pressure induction discharge and the electrodeless plasmotron. Soviet Physics Uspekhi, 1970, vol. 12, pp. 777–791. doi: 10.1070/PU1970v012n06ABEH003972
 

PICTURES

a)                                                                                   b)

Fig. 1. Experimental setup for study of gas discharge: installation diagram (a); installation overall view (b)

a)

b)

c)

d)

e)

f)

 

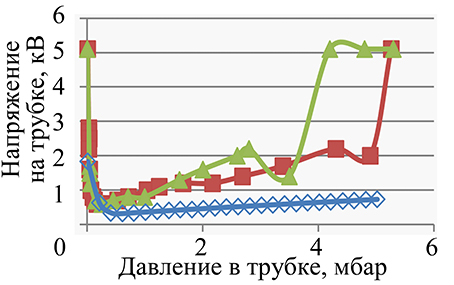
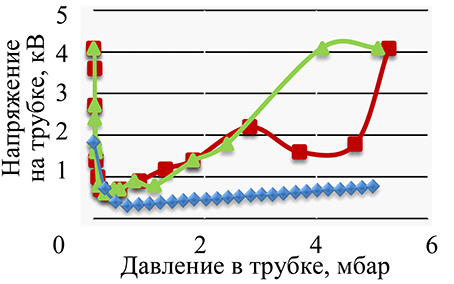
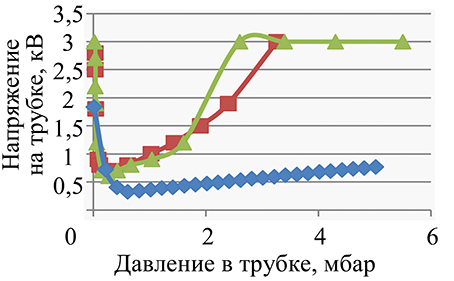
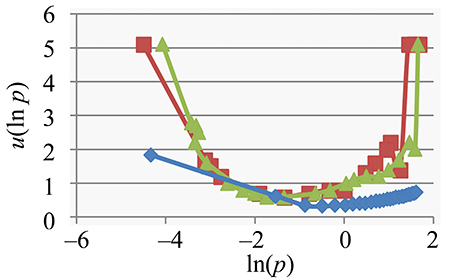
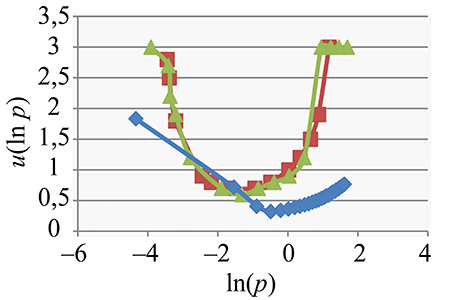
Fig. 2. Measurement results of the dependence of the voltage drop across the discharge gap on the magnitude of the voltage supplying the discharge circuit and air mixture pressure in the discharge gap in cases of tube pumping (triangular markers) and the air mixture puffing (markers in the form of squares) together with numerical simulation results in the framework of semi-empirical approach for modeling gas (markers in the form of lozenges). For the convenience of comparison, the data is shown in linear scale on the pressure axis (at the power voltage of the discharge circuit of 5.1 kV (a); at 4.1 kV (b); at 3 kV (c)) and logarithmic (at power supply voltage of the discharge circuit of 5.1 kV (g); at 4.1 kV (d); at 3 kV (e)), where ln(p) is the logarithm of pressure, and u(ln(p) is the dependence of the voltage drop on the logarithm of pressure

a)

b)

c)

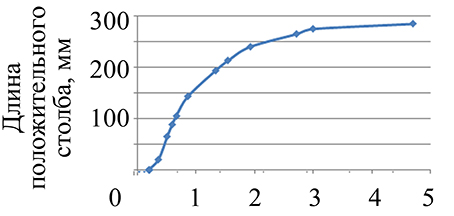
 Fig. 3. Length dependence of glow discharge positive column in an air mixture on the gas pressure at various values of voltage feeding the discharge circuit: U=5.1 kV (a);U=4.1 kV (b); U=3.0 kV (c)

 Fig. 4. The comparison of measurement results of glow discharge electrical parameters in air mixture (1 – at gas puffing, 2 – at exhaust) with the results obtained in the framework of semi-analytical model (3) and hydrodynamic numerical micro models of the discharge in argon (4) and model monatomic gas (5)

Fig. 5. The calculation results of the dependence of voltage drop magnitude on the pressure over the discharge gap for various plasma-chemical models of nonlocal plasma: 1 – experiment;2 – semi-analytical model; 3 – consideration in the framework of the hydrodynamic model of set of reactions (3);4 – consideration of reactions (3) together with the excitation of vibrations and rotations of molecules (4); 5 – additional consideration of inelastic collisions (5), leading to electronic excitation of the molecules; 6 – model taking into account the totality of the processes (3)–(6) for the discharge in the mixture of nitrogen and oxygen

a)

 

b)

Fig. 6. The changes in longitudinal (a) and transverse (b) profiles of ion concentrations for air mixture main component (N2) when accounting for the elementary processes with participation of oxygen:curves 1 – concentrations of N2 +in the discharge in pure nitrogen; curves 2 – concentrations of N2 +in the discharge in air-like mixture with 30% addition of oxygen; curves 3 – concentrations of О2 +in the discharge in air-like mixture

 Fig. 7. The variation results of secondary electron emission coefficient from the cathode in the calculations of the dependence u(p)in the model (3)–(6); curve 1 – experimental data;curve 2 – γ = 0.1; curve 3 – γ = 0.05; curve 4 – γ =0.02; curve 5 – γ = 0.01

Fig. 8. Comparison of experimentally obtained dependences u(p)with results of numerical modeling taking into account reactions (3)–(6) with the specified value γ = 0.02: curves 1 and 2 – experiment and simulation results for U1= 5.1 kV; curves 3 and 4 – experiment and simulation results for U2 = 4.1 kV; curves 5 and 6 – experiment and simulation results for U3 = 3.0 kV

 

a)                                                                                  b)

 

c)                                                                                  d)

e)                                                                                  f)

 

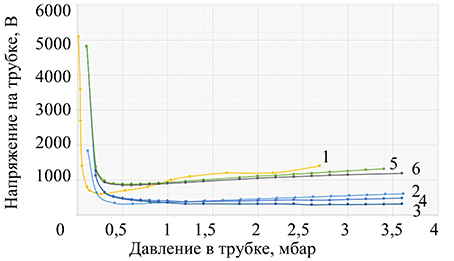
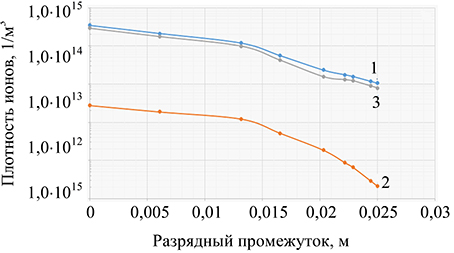
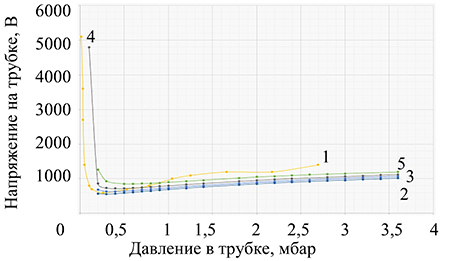
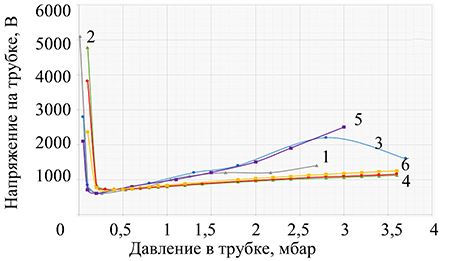
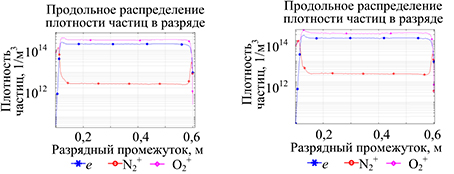
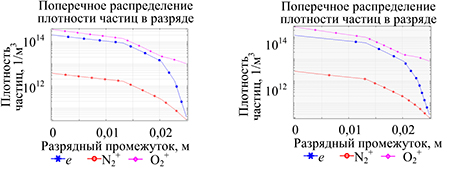
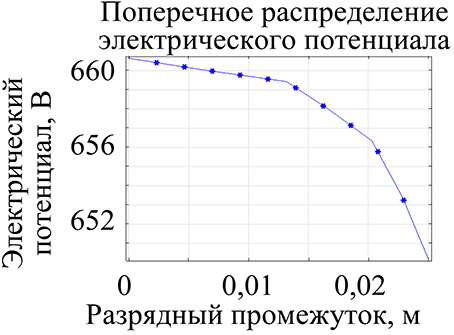
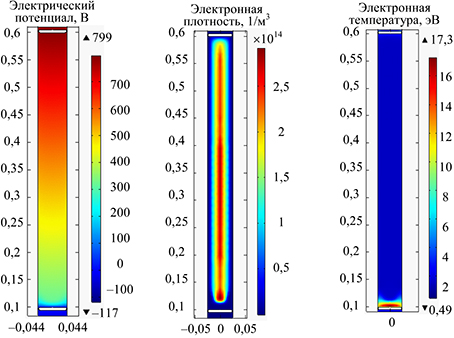
g)

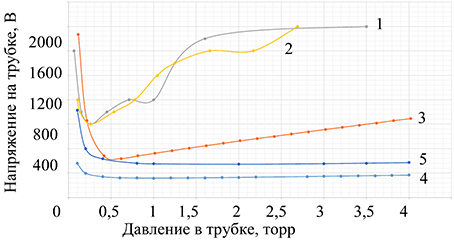
 Fig. 9. The internal parameters of gas discharge in air mixture at lower pressure, obtained in the framework of the model (3)–(6) for voltages equal to 5.1 kV, 4.1 kV and 3 kV: longitudinal distribution of the particle density in category (a)–(c); cross section (g)–(e); transverse distribution of electric potential (f)–(i)

 Fig. 10. Examples of two-dimensional distributions of the discharge internal parameters: electric potential (a); concentration (b), electron temperature (cFig. 9. The internal parameters of gas discharge in air mixture at lower pressure, obtained in the framework of the model (3)–(6) for voltages equal to 5.1 kV, 4.1 kV and 3 kV: longitudinal distribution of the particle density in category (a)–(c); cross section (g)–(e); transverse distribution of electric potential (f)–(i))

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License